******************************************************
one
(1) lateral/curved surface area of Cuboid : 2h(L+b)
Total surface area of Cuboid : 2(Lb+bh+hL)
Volume of Cuboid : L*b*h
Nomenclature : L=Length, b=breadth, h=height
*****************************************
(2) lateral/curved surface area of
Cube : 4a^2
Total surface area of
cube : 6a^2
Volume of a
cube = a^3
nomenclature = a: side of the cube
(3)lateral/curved surface area of
Right prism :perimeter of base * height
Total surface of right Prism :
Lateral surface area + 2(area of the end surface)
Volume of
right prism : area of base * height
(4) Lateral/curved surface area of
regular circular cylinder :2𝝿rh
Total surface area of
regular circular cylinder : 2𝝿(r+h)
Volume of
regular circular cylinder :𝝿*r^2 *h
Nomenclature : r= radius of the base, h=height
(5) lateral/curved surface area of Right pyramid :
1/2(perimeter of base) * Slant height
Total surface area of Right pyramid :
Lateral surfaces area + area of the base
Volume of
right pyramid : 1/3 area of the base * height
(6) Lateral/curved surface area of
right circular cone :𝝿*r*L
Total surface area of
right circular cone : 𝝿r(L+r)
Volume of
right circular cone : 1/3𝝿r^2h
Nomenclature :
r=radius of the base, h=height, L=slant height
(7) lateral/curved surface area of the
Sphere : 4𝝿r^2
Total surface area of the
sphere : 4𝝿r^2
Volume of the
sphere : 4/3𝝿r^3
nomenclature : r= radius
(8) lateral/curved surface area of the
Hemisphere : 2𝝿r^2
Total surface area of the
Hemisphere : 3𝝿r^2
Volume of the
Hemisphere : 2/3𝝿r^3
Nomenclature : r = radius
Example-1) The radius of a conical tent is 7 meters and its height is 10 meters. calculate the length of canvas used in making the tent if width of canvas is 2m. [Use 𝝿=22/7]
sol) Given: radius(r) of conical tent = 7m, Height(h)=10m
.^. So,
the slant height of the cone L^2 = r^2 + h^2
=> L = √r^2 + h^2
=> L = √(7)^2 + (10)^2
=> L = √49 + 100
=> L = √ 149 = 12.2m
Now, surface area of the tent = 𝝿rL
=> 22 * 7 * 12.2
7
=> 268.4 m^2.
Area of canvas used = 268.4m^2
It is given the width(breadth) of the canvas = 2m
we have,
Area = length * Breadth(width)
length = Area
Breadth
=> 268.4
2
=> 134.2m
********************************************************
Example-2). An oil drum is in the shape of a cylinder having the following dimensions : diameter is 2m. and height is 7 meters. The painter charges 3rs/m^2 to paint the drum. Find the total charges to be paid to the painter for 10 drums?
sol) Given : diameter of the (oil drum) = 2m.
radius of cylinder =(d/2) = (2/2) = 1m
Total surface area of
cylinderical drum = 2*𝝿r(r+h)
=> 2 * (22/7) * 1 (1+7)
=> 2 * (22/7) * 8
=> 352 m^2
7
=> 50.28 m^2
So, the total surface area of a drum = 50.28 m^2
painting change per 1m^2 = 3rs
Cost of painting of 10 drums = 50.28 * 3 * 10
=> 1508.40 Rs
*****************************************************
Example-3. A sphere, a cylinder and a cone are of the same radius and same height. Find the ratio of their curved surface areas?
sol) Let "r" be the common radius of a sphere, a cone, and cylinder.
Height of sphere = its diameter = 2r.
Then, the height of the cone = height of cylinder = height of sphere. = 2r.
Let"L" be the slant height of cone = √r^2 +h^2
=> √r^2 + (2r)^2
=> √5r^2
=> r√5
S1 = Curved surface area of sphere = 4𝝿r^2
S2 = Curved surface area of cylinder, 2𝝿rh = 2𝝿r*2r = 4𝝿r^2
S3 = Curved surface area of cone = 𝝿rL = 𝝿r*√5r = √5𝝿r^2
Ratio of curved surface area as
.^. S1 : S2 : S3 = 4𝝿r^2 : 4𝝿r^2 : √5𝝿r^2
=> 4 : 4 : √5
******************************************************
Example-4. A company wanted to manufacture 1000 hemispherical basins from a thin steel sheet. If the radius of hemisphere basin is 21 cm., find the required area of steel sheet to manufacture the above hemispherical basins?
sol) Radius of the hemispherical basin(r) = 21 cm
Surface area of a hemispherical basin = 2𝝿r^2
=> 2 * (22/7) * 21 * 21
=> 2772 cm^2
So, surface area of a hemispherical basin = 2772 cm^2
Hence, the steel sheet required for one basin = 2772 cm^2
Total area of steel required for 1000 basins = 2772 * 1000
=> 2772000 cm^2
converting "cm" into "m"
To convert from square centimeters to square meter either multiply 0.0001 or divide by 10000
Note : One meter or centimeter is a measure of distance whereas we use square meter or square centimeters to measure the area
=> 277.2 m^2
******************************************************
Example-5. A right circular cylinder has base radius 14cm and height 21cm.
Find
(1) Area of base or area of each end.
sol) Area of base(area of each end) 𝝿r^2 = (22/7) *(14)^2
=> 616 cm^2
(2) Curved surface area
sol) Curved surface area = 2𝝿rh
=> 2*(22/7) * 14 * 21
=> 1848cm^2
(3) Total surface area and
sol) TSA = 2 * area of the base +curved surface area.
=> 2 * 616 + 1848
=> 3080 cm^2
(4) Volume of the right circular cylinder.
sol) Volume of cylinder = 𝝿 *r^2*h= area of base * height
=> 616 * 21
=> 12936 cm^3
*********************************************************
Example-6. Find the volume and surface area of a sphere of radius 2.1cm (𝝿 = 22/7)
sol) Radius of sphere(r) = 2.1 cm
Surface area of sphere = 4𝝿r^2
=> 4 * (22/7)*(2.1)^2
=> 4*(22/7)*(21/10)*(21/10)
=> 1386 = 55.44 cm^2
25
Volume of sphere = 4/3*𝝿r^3
=> 4/3 * (22/7)*(2.1)^3
=> 4/3 * (22/7) * 2.1 * 2.1*2.1
=> 38.808 cm^3
*******************************************************
Example-7. Find the volume and the total area of a hemisphere of radius 3.5 cm. (𝝿 = 22/7)
sol) Radius of sphere(r) is 3.5 cm = 7/2 cm
Volume of hemisphere = 2/3*𝝿r^3
=> 2/3 * (22/7) * (7/2)*(7/2)*(7/2)
=> 539 = 89.93 cm^3
6
Total surface area = 3𝝿 r^2
=> 3*(22/7)*(7/2)*(7/2)
=> 231
2
TSA= 115.5 cm^2
***************************************************
Exercise - 10.1
(1) A joker's cap is in the form of right circular cone whose base radius is 7 cm and height is 24 cm. Find the area of the sheet required to make 10 such caps.
sol) Given :- radius(r) = 7 cm, height(h) = 24 cm
Let slant height be "L"
RTP :- Area of the sheet required to make 10 such caps.
We know that
L^2 = h^2 + r^2
L^2 = (24)^2 + ( 7)^2
L^2 = 576 + 49
L^2 = 625
L = 25 cm
Curved Surface Area of 1 jokers cap = Pi * r * L
=> 22 *
=> 22 * 25
=> 550 cm^2
(*) Curved Surface Area of 10 jokers cap = 10 * 550
=> 5500 cm^2
*********************************************
(2) A sports company was ordered to prepare 100 paper cylinders for shuttle cocks. The required dimensions of the cylinder are 35 cm length/ height and its radius is 7 cm. Find the required area of thin paper sheet needed to make 100 cylinders?
sol) Given :- radius(r) = 7 cm & length/height = 35 cm
Total surface area of the cylinder = 2 * Pi * rh
=> 2 * 22 * (
7
=> 1540 cm^2
Required paper sheet for 100 such cylinders = 100 * 1540
=> 154000 cm^2
*************************************************
(3) Find the volume of right circular cone with radius 6 cm, and height 7 cm.
sol) given :- r = 6 cm and h = 7 cm
Volume of cone :-
=> 1 * Pi * r^2 * h
3
=> 1 * 22 * 6 *
= 264 cm^3
*****************************
(4) The lateral surface area of a cylinder is equal to the curved surface area of a cone. If the radius be the same, find the ratio of the heights of the cylinder and slant height of the cone.
sol) Let the radius be same = r
The lateral surface of a cylinder = 2 * Pi * r * h
Curved surface area of a cone = Pi * r * L
Curved surface area of a cone = The lateral surface of a Cylinder
so,
Pi * r * l = 2 * Pi * r * h
=> L = 2h
=> h = 1
L 2
So, the ratio is 1 ; 2
******************************************
(5) A self help group wants to manufacture joker's caps ( conical caps) of 3 cm, radius and 4 cm, height. If the available color paper sheet is 1000 cm.sq, Than how many caps can be manufactured from that paper sheet.?
sol) Given :- r = 3 cm, and h = 4 cm
Slant height(L) :-
L^2 = r^2 + h^2
L^2 = (3)^2 + ( 4)^2
L^2 = 9 + 16
L^2 = 25
L = 5 cm
Surface area of one such conical cap = Pi * r * L
=> 22 * 3 * 5
7
=> 330
7
=> 47.14 cm^2
Given :- Total area of sheet = 1000 cm^2
No. of caps that can be made = 1000
47.14
=> 21.21 caps
****************************************
(6) A cylinder and cone have bases of equal radii and are of equal heights. Show that their volumes are in the ratio of 3 : 1.
sol) Let the radii = r and height = h
The volume of cylinder, V1 = Pi * r^2 * h -------(1)
The volume of cone, V2 = 1 * Pi * r^2 * h -----(2)
3
Now, 1 divide (2)
v1 = Pi * r^2 * h
v2 = 1 * Pi * r^2 * h
3
v1 = 3 * Pi * r^2 * h
v2 = Pi * r^2 * h
v1 = 3
v2 = 1
So, the volume of cylinder and volume of cone are in the ratio 3 : 1
*********************************************************
(7) A solid iron rod has a cylinder shape. Its height is 11 cm, and base diameter is 7 cm, Then find the total volume of 50 rods?
sol) given :- h = 11 cm
Base diameter = 7 cm
.^. r = 7 = 3.5 cm
2
Volume of one rod = Pi * r^2 * h
=> 22 * 3.5 * 3.5 * 1
7
=> 423.5 cm^3
Total volume of 50 such rods :- 50 * 423.5
=> 21175 cm^3
*************************************
(8) A heap of rice is in the form of a cone of diameter 12 m, and height 8 m. Find its volume? How much canvas cloth is required to cover the heap? ( Use Pi = 3.14)
sol) Given :- Diameter(d) = 12 m
Radius(r) = 12 /2 = 6 m
height (h) = 8 m
Volume :- 1 * Pi * r^2 * h
3
=> 1 * 22 * (6)^2 * 8
3 7
=> 301.71 cm^3
Cloth required to cover up the heap = Curved surface area of the cone
=> Pi * r * L
=> 22 * 6 * 10
7
=> 188.6cm^2
****************************************************
(9) The curved surface area of a cone is 4070 cm.sq and its diameter is 70 cm. What is its slant height?
sol) Given :- Diameter = 70 cm
Radius(r) = 70 = 35
2
curved surface area = 4070
RTP :- Slant height (L)
We have
Curved surface area = Pi * r * L
4070 = 22 *
4070 = L
110
Slant height ( L) = 37 cm
*********************************************************************************
Example-8. A right triangle, whose base and height are 15 cm, and 20 cm. respectively is made to revolve about its hypotenuse. Find the volume and surface area of the double cone so formed (Use 𝝿 = 3.14)
sol) Let ABC be the right angled triangle such that
AB = 15cm and AC = 20cm
Using Pythagoras theorem in △ABC we have
BC^2 = AB^2 + AC^2
BC^2 = (15)^2 + (20)^2
BC^2 = 225 + 400 = 625
BC = √625 = 25 cm.
Let OA = x and OB = y.
In triangles ABO and ABC, we have ∠BOA =∠BAC and ∠ABO = ∠ABC
So, by angle-angle-criterion of similarity, we have △BOA~△BAC
.^., BO = OA = BA
BA AC BC
=> y = x = 15
15 20 25
=> y = x = 3
15 20 5
=> y = 3 * 15 and x = 3 * 20
5 15
=> y = 9 and x = 12.
Thus, we have
OA = 12 cm and OB = 9cm
When the ABC is revolved about the hypotenuse, we get a double cone as shown in figure.
Volume of the double cone = volume of the cone CAA' + volume of the cone BAA'
=> 1 𝝿(OA)^2 * OC + 1 𝝿(OA)^2 * OB
3 3
=> 1 𝝿*(12)^2 * 16 + 1 𝝿*(12)^2 *9
3 3
=> 1 𝝿 * 144 (16 +9)
3
=> 1 * 3.14 * 144 * 25 cm^3
3
=3768 cm^3
Surface area of the double cone = (Curved surface area of cone CAA') + (Curved surface area of cone BAA')
=> (𝝿*OA*AC) + (𝝿*OA*OB)
=> (𝝿*12*20) + (𝝿 * 12 * 15) cm^2
=> 420 𝝿 cm^2
=> 420 * 3.14 cm^2
=> 1318.8 cm^2
******************************************************
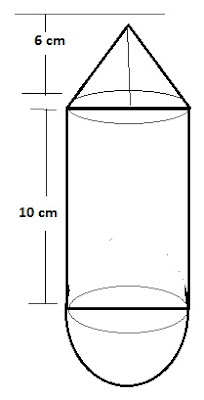
Example-9. A wooden toy rocket is in the shape of a cone mounted on a cylinder as shown in the adjacent figure. The height of the entire rocket is 26 cm, while the height of the conical part is 6cm.
The base of the conical position has a diameter of 5cm, while the base diameter of the cylindrical portion is 3cm. If the conical portion is to be painted orange and the cylindrical portion is to be painted yellow,
find the area of the rocket painted with each of these color
( Take 𝝿 = 3.14)
sol) Let 'r' be the radius of the base of the cone and its slant height be 'L'. Further, let r1 be the radius of cylinder and h1 be its height
We have,
r = 2.5 cm., h = 6 cm.
r1 = 1.5 cm. h1 = 20 cm.
Now, L = √r^2 + h^2
=> L =√ (2.5)^2 + (6)^2
=> L = √6.25 + 36
=> L = √42.25 = 6.5
Now, area to be painted orange = Curved surface area of the cone
=> 𝝿rL
=> 3.14 *(2.5*6.5)
=> 51.025 cm^2
Area to be painted yellow = Curved surface area of the cylinder + Area of the base of the cylinder
=> 2𝝿r1h1 + 𝝿r1^2
=> 𝝿r1(2h1 +r1)
=> 3.14 * 1.5( 2 * 20 + 1.5) cm^2
=> 3.14 * 1.5 * 41.5 cm^2
=> 4.71 * 41.5 cm^2
=> 195.465 cm^2
.^. ,area to be painted yellow = 195.465 cm^2
******************************************************
Exercise - 10.2
(1) A toy is in the form of a cone mounted on a hemisphere. The diameter of the base and the height of the cone are 6 cm and 4cm respectively. Determine the surface area of the toy.{ use Pi = 3.14]
sol) Given :- diameter of cone = 6 cm
radius ( r) of cone = 6 / 2 = 3 cm
height(h) = 4 cm.
RTP :- Surface area of the toy
Surface area of the toy = surface area of the cone + surface area of the hemisphere
Now,
Slant Height (L) :-
L^2 = r^2 + h^2
L^2 = (3)^2 + (4)^2
L^2 = 9 + 16
L^2 = 25
L = 5
Curved surface area of the cone = Pi * r * L
=> 3.14 * 3 * 5
=> 47.1 cm^2 -----(1)
Curved surface area of the hemisphere = 2 * Pi * r^2
=> 2 * 3.14 * (3)^2
=> 56.52 cm^2 -------(2)
Now,
Total surface area of the toy = (1) + (2)
=> 47.1 + 56.62
=> 103. 72 cm^2
**********************************************
(2)A solid is in the form of a right circular cylinder with a hemisphere at one end and a cone at the other end. The radius of the common base is 8 cm, and the heights of the cylindrical and conical portions are 10 cm and 6 cm respectively. Find the total surface area of the solid [ use Pi = 3.14]
sol) Given :- Common radius of cylinder, hemisphere, and cone = 8 cm
Height of cylinder = 10 cm
Height of cone = 6 cm
Pi = 3.14
We know that
T.S.A of the solid = S.A hemisphere + S.A cylinder + S.A cone
Slant height (L) :-
L^2 = r^2 + h^2
L^2 = (8)^2 + (6)^2
L^2 = 100
L = 10
(a) S.A of hemisphere = 2*Pi*r^2
=> 2 * 3.14 * 64
=> 401.92 cm^2 ------------(1)
(b) Surface area of cylinder = 2 * Pi * r * h
=> 2 * 3.14 * 8 * 10
=> 502.4 cm^2 -----------(2)
(c) Surface area of the cone = Pi * r * L
=> 3.14 * 8 *6
=> 150.72 cm^2 ------------------(3)
T.S.A of the solid = (1) + (2) + (3)
=> 401.92 + 502.4 + 150.72
=> 1055.04 cm^2
***************************************
(3) A medicine capsule is in the shape of a cylinder with two hemispheres stuck to each of its ends. The length of the capsule is 14 mm. and the width is 5 mm. Find its surface area.
sol) Given :- Length of cylinder, h = 14 mm
width of cylinder = 5 mm
.^. radius of cylinder, r = 2.5 mm
There are two hemisphere attached at each end of the capsule
The S.A cylinder = S.A hemi.1 + S.A hemi.2 + S.A of cylinder
=> 2* Pi * r^2 + 2 * Pi * r^2 + 2 * Pi *rh
=> 4*Pi*r^2 + 2 * Pi * r * h
=> 4 * 3.14 * 2.5 * 2.5 + 2 * 3.14 * 2.5 * 14
=>298.3 mm^2 or 300 mm^2 ( approx)
***************************************
(4) Two cubes each of volume 64 cm^3 are joined end to end together. Find the surface area of the resulting cuboid.
sol) Given :- Volume of each cube = 64 cm^3
i.e, (side)^3 = 64 cm^3
=> a^3 = 64 cm^3
=> a^3 = (4)^3
=> a = 4
So, side of a cube a = 4 cm
Since two cubes are joined end to end together there each side "a" and "a" gives the total length of the cuboid.
Length of cuboid (a+a) = 4 + 4 = 8 cm
Breadth (b) = a = 4 cm
Height (h) = a = 4 cm
Formula :-
Area of cuboid = 2 ( lb + bh + hl)
=> 2( 8 * 4 + 4*4 + 4*8)
=>2( 32 + 16 + 32)
=> 2(80)
=> 160 cm^2 is the area of cuboid
***********************************
(5) A storage tank consists of a circular cylinder with a hemisphere stuck on either end. If the external diameter of the cylinder be 1.4 m and its length be 8 m., find the cost of painting it on the outside at rate of 20Rs per m.sq
sol) Given :- Diameter = 1.4 m
radius(r) =
2
Height(h) = 8 m
cost :- 20Rs per.m.sq
Total area of the storage tank = C.S.A of Hemi + C.S.A of cylinder
C.S.A of Hemi = 2 * Pi * r^2
=> 2 * 3.14 * ( 0.7)^2
=>3.08 m^2 ------------------(1)
(b) C.S.A of Cylinder :- 2* Pi * r * h
=> 2 * 3.14 * 0.7 * 8
=> 35.2 m^2 --------------(2)
Total area of the storage tank = (1) + (2)
=> 35.2 + 3.08
=> 38.28 m^2
Total cost of painting = 20 * 38.28 = 765.6 Rs
*********************************************
(6) A sphere, a cylinder and a cone have the same radius and same height. Find the ratio of their volumes. [ Hint : Diameter of the sphere is equal to the heights of the cylinder and the cone.]
Height of the sphere = its diameter = 2r
Then, the height of the cone = height of cylinder = height of sphere = 2r
Slant height(L) :-
L^2 = r^2 + h^2
L^2 = r^2 + (2r)^2
L^2 = r^2 + 4r^2
L^2 = 5r^2
L = r_/5
Now,
C.S.A of sphere (s1) = 4 * Pi * r^2
C.S.A of cylinder ( s2) = 2 * Pi * r * h
=> 2 * Pi * r * 2r = 4 Pir^2
C.S.A of cone (s3) = Pi * r * L
=> Pi * r * r_/5 = _/5 Pi*r^2
Ratio of C.S.A :-
s1 : s2 : s3 = 4 : 4 : _/5
****************************************
(7) A hemisphere is cut out from one face of a cubical wooden block such that the diameter of the hemisphere is equal to the length of the cube. Determine the surface area of the remaining solid.
sol) Here, diameter of the hemisphere is equal to the length of the cube
So, diameter = side of cube = a
Here, base of hemisphere would not be included in the total solid area of wooden cube.
S.A of solid = Area of cube + C.S.A of hemi - Base area of hemisphere
(a) Area of cube :-
Here, side = a
Area of cube = 6 (side)^2 = 6 a^2
(b) Curved surface area of hemisphere :-
Diameter of hemisphere = a
Hence, radius (r) = Diameter = a
2 2
Formula :-
Curved surface area of hemisphere = 2 * Pi * r^2
=> 2 * Pi * (a)^2
2
=>
2
(c) Base area of hemisphere :-
Base of hemisphere is a circle with radius = radius of hemisphere = r = a/2
Base area of hemisphere = Pi * r^2
=> Pi * ( a ) ^2
2
=> Pi * a^2
4
=> Pi * a^2
4
Now,
Surface area of solid = Area of cube + C.S.A of hemi - Base area of hemi
=> 6a^2 + (Pi * a^2) - (Pi * a^2)
2 4
=> 6a^2 + (2*pi*a^2 - Pi*a^2)
4
=> 6a^2 + Pi * a^2
4
=> a^2 ( 6 + Pi)
4
=> a^2 ( 24 + Pi )
4
=> a^2 (6 + Pi ) cm^2
4
*************************************************
(8) A wooden article was made by scooping out a hemisphere from each of a solid cylinder as shown in the figure. If the height of the cylinder is 10 cm, and its base is of 3.5 cm, find the total surface area of the article.
sol) Given :- h= 10 cm and r = 3.5 cm
RTP :- T.S.A of the article
(a) C.S.A of cylinder = 2 * Pi * r * h
=> 2 * 3.14 * 3.5 * 10 = 219.8
(b) C.S.A of two hemisphere = 2 * (2 * Pi * r^2)
=> 2(2 * 3.14 * (3.5)^2)
=> 153.86
T.S.A = (1) + (2)
=> 219.8 + 153.86
=> 374 cm^2
*********************************************************************************
Note : In calculating the surface area of combination of solids, we cannot add the surface areas of the two solids because some part of the surface areas disappears in the process of joining them.
However, this will not be the case when we calculate the volume. The volume of the solid formed by joining two basic solids will actually be the sum of the volumes of the constituents
Example-10. A solid toy is in the form of a right circular cylinder with hemispherical shape at one end and a cone at the other end. Their common difference is 4.2 cm and the height of the cylindrical and conical portions are 12cm and 7cm respectively. Find the volume of the solid toy. ( Use 𝝿 = 22/7)
sol) Let height of the conical portion(h1) = 7cm
The height of cylindrical portion(h2) = 12cm
Radius(r) = 4.2/2 = 2.1 = 21/10 cm
Volume of the solid toy = Volume of the Cone + Volume of the cylinder + Volume of the Hemisphere
= 1/3 𝝿r^2*h1 + 𝝿r^2*h2 + 2/3*𝝿r^3
= 𝝿r^2[1/3*h1 + h2 + 2/3r]
= 22/7 *(21/10)^2 *[ (1/3) *7 + 12 +(2/3)*(21/10]
= 22/7 * 441/100 * [ 7/3 + 12/1 + 7/5 ]
= 22/7 * 441/100*[ 35 + 180 + 21]
15
= 22 * 441 * 236
7 100 15
= 27258
125
= 218.064 cm^3
*******************************************************
Example-11. A cylindrical container is filled with ice-cream whose diameter is 12cm and height is 15cm. the whole ice-cream is distributed to 10 children in equal cones having hemispherical tops. If the height of the conical portion is twice the diameter of its base, find the diameter of the ice-cream cone.
sol) Let the radius of the base of conical ice-cream = x cm
.^. diameter = 2x cm
Then,
The height of the conical ice-cream = 2(diameter)
= 2(2x) = 4x cm
Volume of ice-cream cone = Volume of conical portion + volume of hemispherical portion
= 1 𝝿r^2h + 2 𝝿r^3
3 3
= 1 𝝿 x^2(4x) + 2 𝝿 x^3
3 3
= 4𝝿x^3 + 2𝝿 x^3
3
= 6𝝿 x^3
3
= 2𝝿 x^3 cm^3
Diameter of cylindrical container = 12 cm
Its height(h) = 15 cm
.^. Volume of cylindrical container = 𝝿 r^h
= 𝝿 (6)^2* 15
= 540𝝿 cm^3
Number of children to whom ice-cream is given = 1
Volume of cylindrical container = 10
Volume of one ice-cream cone
=> 540𝝿 = 10
2𝝿 x^3
2𝝿 x^3 * 10 = 540*𝝿
x^3 = 540 = 27
2 * 10
x^3 = 27
x^3 = 3^3
x = 3
.^. Diameter of ice-cream cone 2x=2(3) = 6cm
********************************************************
Example-12. A solid consisting of a right circular cone standing on a hemisphere, is placed upright in a right circular cylinder full of water and touched the bottom.
Find the volume of water left in the cylinder, given that the radius of the cylinder is 3 cm, and its height is 6cm. The radius of the hemisphere is 2cm, and the height of the cone is 4cm.
[ Take 𝝿 = 22/7 ]
sol) In the figure drawn here,
ABCD is a cylinder and LMN is a Hemisphere.
OLM is a cone. We know that where a solid consisting of a cone and hemisphere is immersed in the cylinder full of water, then some water equal to the volume of the solid, is displaced.
(*) Volume of Cylinder = 𝝿 r^2h
= 𝝿 *(3)^2 * 6
= 54 𝝿 cm^3
(*)Volume of hemisphere = 2/3𝝿 r^3
= 2/3 *𝝿 *(2)^3
= 16 𝝿 cm^3
3
(*)Volume of Cone = (1/3)𝝿 r^2*h
= 1/3 * 𝝿 * (2)^2 * 4
= 16/3 𝝿 cm^3
(*) Volume of cone + hemisphere =(16/3)𝝿 +(16/3)𝝿
= 32 𝝿
3
Volume of water left in cylinder = Volume of Cylinder - volume of Cone and hemisphere
= Volume of cylinder - (32/3)𝝿
= 54𝝿 - 32𝝿
3
= 162𝝿 - 32𝝿
3
= 130 𝝿
3
= 130 * 22
3 7
= 2860
21
= 136.19 cm^3
********************************************************
Example-13. A cylindrical pencil is sharpened to produce a perfect cone at one end with no over all loss of its length. The diameter of the pencil is 1cm and the length of the conical portion is 2cm. Calculate the volume of the shavings. Give your answer correct to two places if its in decimal
[ use 𝝿 = 355 }
113
sol) Diameter of the pencil = 1 cm
so, radius of the pencil(r) = 0.5 cm
length of the conical portion =h= 2cm
Volume of showings = volume of cylinder of length 2cm and base radius 0.5 cm - volume of the cone formed by this cylinder.
= 𝝿 r^h - (1/3)𝝿 r^h
= (2/3)𝝿 r^2* h
= 2 * 355 * (0.5)^2 * 2 cm^3
3 113
= 1.05 cm^3
********************************************************
Exercise - 10.3
(1) An iron pillar consists of a cylindrical portion of 2.8 m, height and 20 cm, in diameter and a cone of 42 cm, height surrounding it. Find the weight of the pillar of 1 cm.cube of iron weighs 7.5 g.
sol) Given :- Diameter = 20 cm
radius(r) = 20/2 = 10 cm
Height of the cone = 42 cm
Height of the cylinder = 2.8 cm = 280 cm { 100cm = 1m)
(a) Volume of cone = 1/3 * Pi * r^2 * h
=> 1/3 * 3.14 * (10)^2 * 42
=> 4396 cm^3 ----------(1)
(b) Volume of cylinder = Pi * r^2 * h
=> 3.14 * (10)^2 * 280 cm
=> 87,920 cm^3 ---------(2)
Volume of the iron pillar = (1) + (2)
=> 4396 + 87920
=> 92316 cm^3
Weight of the iron pillar = 92,316 * 7.5
=> 692,370 g
=> 692 . 370 kg { 1000g = 1kg)
*****************************************
(2) A toy is made in the form of hemisphere surmounted by a right cone whose circular base is joined with the plane surface of the hemisphere. The radius of the base of the cone is 7 cm, and its volume is 3/2 of the hemisphere. Calculate the height of the cone and the surface area of the toy correct to 2 places of decimal ( Take Pi = 3(1/7)]
sol) Given :- radius(r) = 7 cm
The volume of the cone = 3 /2 ( volume of hemisphere)
Now,
1 * Pi * r^2 * h = 3 [ 2 * Pi * r^3 ]
3 2 3
1 * Pi * r^2 * h = Pi * r^3
3
Pi * r^2 * h = 3Pi* r^3
h = 3r
h = 3(7)
h = 21 cm
Slant Height(L) :-
L^2 = r^2 + h^2
L^2 = (7)^2 + (21)^2
L^2 = 49 + 441
L^2 = 490
L = _/49 * 10
L = 7_/10 cm [ _/10 = 3.16 ]
L = 22.13
Surface area of the toy = C.S.A of cone + Hemisphere
=> 2* Pi * r^2 + Pi * r * L
=> Pi [ 2 * (7)^2 + 7 * 22.13 ]
=> Pi [ 252.91]
=> 252.91 * 3.14
=> 794.13 cm^2
*******************************************
(3) Find the volume of the largest right circular cone that can be cut out of a cube whose edge is 7 cm.
sol) Given :- Height of the cone = 7 cm
We know in cube all sides are equal height and diameter will be same
.^. Diameter of the cone = 7 cm
Radius(r) = 3.5 cm
Volume of the cone :-
=> 1 * Pi * r^2 * h
3
=> 1 * Pi * (3.5)^2 * 7
3
=> 1/3 * 3.14 * (3.5)^2 * 7
=> 89. 75 cm^3
*****************************************
(4) A cylinderical tub of radius 5cm and length 9.8 cm is full of water. A solid in the form of right circular cone mounted on a hemisphere is immersed into the tub. The radius of the hemisphere is 3.5 cm and height of cone outside the hemisphere is 5 cm. Find the volume of water left in the tub ( Take Pi = 22/7)
sol) Given :- Radius of cylinder = 5 cm
Height of cylinder = 9.8 cm
(a) Volume of water in the tub = Pi * r^2 * h
=> 3.14 * (5)^2 * 9.8 cm^3
=> 769.3 cm^3 ------(1)
From figure we can see
Volume of toy = Volume of hemisphere + Volume of cone
(1) Finding volume of hemisphere :-
Radius of hemisphere (r1) = 3.5 cm
.^. Volume of hemisphere = 2/3 * Pi * r1^3
=> 2/3 * 3.14 * (3.5)^3 cm^3
=> 89.75 cm^3 ----------(2)
(2) Volume of cone :-
Radius of cone (r) = 3.5 cm
height of cone (h) = 5 cm
.^. Volume of cone = 1/3 * Pi * r^2 * h
=> 1/3 * 3.14 * ( 3.5)^2 * 5
=> 64.10 cm^3 ------------------(3)
Now,
Volume of toy = Volume of hemisphere + Volume of cone
=> 89.75 + 64.10
=> 153. 85 cm^3
Now
Volume of water left in the tank = Volume of water in the tub -- Volume of Toy
=> 769.3 - 153.85
=> 615.45 cm^3
******************************************************************
sol) Given :- Height of the cylinder = 10
Diameter of cylinder = 7 cm
height of the cone = 4 cm
radius of cone = 3 cm
(a) Volume of the cylinder = Pi * r^2 * h
=> 3.14 * (3.5)^2 * 10
=> 385 cm^3
(b) Volume of cone = 1/3 * Pi * r^2 * h
=> 1/3 * 3.14 * (3)^2 * 4
=> 37.67 cm^3
Now
Volume of remaining solid = volume of cylinder -- 2*volume of cone
=> 385 -- 2( 37.67)
=> 309.66 cm^3 is the volume of remaining solid
**********************************************
(6) Spherical Marbles of diameter 1.4 cm, are dropped into a cylindrical beaker of diameter 7 cm, which contains some water. Find the number of marbles that should be dropped in to the beaker, so that water level rises by 5.6 cm.
sol)Given :- Diameter of marble = 1.4 cm
radius(r) of marble = 1.4 / 2 = 0. 7 cm
diameter of beaker = 7 cm
radius(r) of beaker = 7/2 = 3.5 cm
water level rises by = 5.6 cm
Volume of 1 marble = 4/3 * Pi * r^3
=> 4/3 * 3.14 * ( 0.7)^3
=> 1.43 cm^3
Let the no.of the marbles dropped = x
Volume of "x" marbles = volume of water displaced in the cylinder
=> 4/3 * Pi * r^3 = Pi * r^2 * h
=> (1.43) * x = 3.14 * (3.5)^2 * 5.6
=> (1.43) * x = 215.40
=> x = 215.40
1.43
=> x = 150 marbles should be dropped.
**********************************
(7) A pen stand is made of wood in the shape of cuboid with three conical depressions to hold the pens. The dimensions of the cuboid are 15cm by 10 cm by 3.5 cm. The radius of each of the depression is 0.5 cm and the depth is 1.4 cm. Find the volume of wood in the entire stand.
sol) Given :- len = 15 cm, breadth = 10 cm, Height = 3.5 cm
radius(r) = 0.5 cm
depth or height of cone = 1.4 cm
(a) volume of cuboid = L * B * H
=> 15 * 10 * 3.5 = 525 cubic cm
(b) Volume of cone = 1/3 * Pi * r^2 * h
=> 1/3 * 3.14 * (0.5)^2 * 1.4
=>0.36 cm^3
Volume of wood = Volume of cuboid -- 6 * volume of cone
=> 525 -- 6 * (0.36)
=> 522.84 cubic m.
*************************************************
Example-14. A cone of height 24cm and radius of base 6 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the radius of the sphere.
sol) Volume of cone = (1/3) * 𝝿 * 6 * 6 * 24 cm^3
If 'r' is the radius of the sphere, then its volume is (4/3)*𝝿*r^3
Since the volume of clay in the form of the cone and the sphere remains the same, we have
4*𝝿 *r^3 = 1 *𝝿 * 6 * 6 * 24
3 3
r^3 = 3 * 3 *24 => 3*3*3*8
r^3 = 3^3 * 2^3
r = 3*2 = 6cm is the radius of the sphere
*******************************************************
Example-15. The diameter of the internal and external surfaces of a hollow hemispherical shell are 6cm and 10cm respectively. It is melted are recast into a solid cylinder of diameter 14cm, Find the height of the cylinder.
sol) Radius of Hollow hemispherical shell = 10/2 = 5cm =R
internal radius of hollow hemispherical shell = 6/2 =3cm = r
volume of hollow hemispherical shell = External volume - Internal volume
= 2 𝝿R^3 - 2 𝝿r^3
3 3
= 2 𝝿(R^3 - r^)
3
= 2 𝝿((5)^3 - (3)^3)
3
= 2 𝝿(125 - 27)
3
= 2 𝝿 * 98 cm^3
3
= 196𝝿 cm^3 ------(1)
3
Since, this hollow hemispherical shell is melted and recast into a solid cylinder. So their volumes must be equal.
Diameter of cylinder = 14 cm.(Given)
So, radius of cylinder = 7 cm.
Let the height of cylinder = h
.^. volume of cylinder = 𝝿r^2*h
= 𝝿 * 7 * 7 * h cm^3
= 49𝝿h cm^3 -------(2)
According to given condition
volume of Hollow hemispherical shell = volume of solid cylinder
from equation(1) & (2)
196𝝿 = 49𝝿h
3
h = 196 = 4 cm
3*49 3
Hence, height of the cylinder = 1.33 cm.
********************************************************
Example-16. A hemispherical bowl of internal radius 15cm. contains a liquid. The liquid is to be filled into cylindrical bottles of diameter 5 cm, and height 6 cm. How many bottles are necessary to empty the bowl?
sol) Volume of hemisphere = (2/3)𝝿r^3
internal radius of hemisphere r = 15cm.
.^. volume of liquid contained in hemisphere bowl
= (2/3) 𝝿(15)^3 cm^3
= 2250 𝝿 cm^3
This liquid is to be filled in cylindrical bottles and the height of each bottle(h) = 6 cm.
Radius of cylindrical bottle(R) = (5/2) cm.
.^. Volume of 1 cylindrical bottle = 𝝿*R^2*h
= 𝝿 * (5/2)^2 * 6
= 𝝿 * (25/4) * 6cm^3
= 75 𝝿 cm^3
2
Number of cylindrical bottles required =
= Volume of hemispherical bowl
Volume of 1 cylindrical bottle
= 2250 𝝿
(75/2) 𝝿
= 2 * 2250 = 60
75
*******************************************************
Example-17, The diameter of a metallic sphere is 6cm. It is melted and drawn into a wire having diameter of the cross section as 0.2 cm. Find the length of the wire.
sol) We have, diameter of metallic sphere = 6cm
.^. Radius of metallic sphere = 3 cm
Also, we have,
Diameter of cross - section of cylindrical wire = 0.2 cm
Radius of cross section of cylinder wire = 0.1 cm
Let the length of wire be "L" cm.
Since the metallic sphere is converted into cylindrical shaped wire of length "h" cm.
.^. Volume of the metal used in wire = Volume of the sphere
𝝿 * (0.1)^2 * h = (4/3) * 𝝿 *(3)^3
𝝿 * (1/10)^2 *h = (4/3) *𝝿*27
𝝿* (1/100) *h = 36𝝿
h = 36𝝿 * 100 cm
𝝿
h = 3600 cm = 36 m.
.^. , the length of the wire is 36m.
*******************************************************
Example-18. How many spherical balls can be made out of a solid cube of lead whose edge measures 44 cm and each ball being 4 cm, in diameter.
sol) Side of lead cube = 44 cm.
Radius of spherical ball = (4/2) cm = 2 cm.
Now volume of spherical bullet = (4/3)𝝿r^3
= (4/3) * (22/7) * (2)^3 cm^3
= (4/3) * (22/7) * 8 cm^3
Volume of "x" special bullet = (4/3) * (22/7) *8 * x cm^3
It is clear that volume of "x" spherical bullets = Volume of lead cube
= (4/3) * (22/7) * 8 * x = (44)^3
= (4/3) * (22/7) * 8 * x = 44 * 44 * 44
= x = 44 * 44 * 44 * 3 * 7
4 * 22 * 8
x = 2541
Hence, total number of spherical bullets = 2541.
******************************************************
Example-19. A women self help group(DWACRA) is supplied a rectangular solid(cuboid shape) of wax with diameter 66 cm, 42 cm, 21 cm, to prepare cylindrical candles each 4.2 cm in diameter and 2.8 cm of height. Find the number of candles.
sol) Volume of wax in the rectangular solid = L*b*h
= ( 66 * 42 *21) cm^3
Radius of cylindrical candle = (4.2/2) cm = 2.1 cm.
Height of cylindrical candle = 2.8 cm.
Volume of candle = 𝝿r^2*h
= (22/7) * (2.1)^2 * 2.8
Volume of 'x' cylindrical wax candles =
= (22/7) *(2.1)*(2.1)*(2.8) * x
Volume of 'x' cylindrical candles = volume of wax in rectangular shape
.^. (22/7) *(2.1)*(2.1)*(2.8) *x = 66 * 42 * 21
x = 66 * 42 * 21 * 7
22*(2.1)*(2.1)*(2.8)
x = 1500
Hence, the number of cylindrical wax candles = 1500.
*******************************************************
Exercise - 10.4
(1) A metallic sphere of radius 4.2 cm, is melted and recast into the shape of a cylinder of radius 6 cm. Find the height of the cylinder.
sol) Given :- radius(r) of sphere = 4.2 cm
radius(r) of cylinder = 6 cm
Since sphere is melted into a cylinder,
So, volume of sphere == volume of cylinder
metallic sphere melted =====> shape of cylinder
(a) Volume of sphere :-
=> 4/3 * Pi * r ^3
=> 4/3 * 3.14 * (4.2)^3
=> 310.18
(b) Volume of cylinder :-
=> Pi * r^2 * h
=> 3.14 * (6)^2 * h
=> 113.04 h
Now,
volume of sphere == volume of cylinder
=> 310.18 = 113.04 * h
=>310.18 = h
113.04
h = 2.74 cm is the height of cylinder.
**********************************
sol)
Since 3 spheres are melted to form one new sphere
volume of 3 old sphere = volume of new sphere
Volume of 1st + 2nd + 3rd sphere = Volume of new sphere
Now,
(a) Volume of 1st sphere, :-
Radius(r1) = 6 cm
=> 4/3 * Pi * (r1)^3
=> 4/3 * 3.14 * (6)^3
=> 904 . 31 -----------------(1)
(b) Volume of 2nd sphere :-
Radius (r2) = 8 cm
=> 4/3 * 3.14 * (8)^3
=> 2143.57 ---------------(2)
(c) Volume of 3rd sphere :-
Radius (r3) = 10 cm
=> 4/3 * 3.14 * (10)^3
=> 4186.66 -----------(3)
now,
Volume of new sphere = 904.31 + 2143.57 + 4186.66
4/3 * 3.14 * r^3 =7234.54
r^3 =
4.186
r^3 = 1728
r^3 = (12)^3
r = 12 is the radius of resulting sphere
************************************
(3) A 20m deep well with diameter 7m, is dug and the earth from digging is evenly spread out to form a platform 22 m, by 14 m. Find the height of the platform.
sol) Given :- well height (h) = 20m
diameter of well = 7m
radius(r) of well = 7/2 = 3.5 m
RTP :- Height of the platform
Here, shape of well is in form of cylinder
Volume of cylinder :-
=> Pi * r^2 * h
=> 3.14 * (3.5)^2 * 20
=> 770 m^3 --------(1)
Also
Given :- length of platform (L) = 22 m
breadth (b) = 14 m
Here, shape of platform will be in shape of cuboid
Volume of cuboid :-
=> L * b * h
=> 22 * 14 * h
=> 308 h --------(2)
Now,
Volume of cylinder == Volume of cuboid
770 = 308 * h
308
2.5 = h is the height of the platform
**********************************
(4) A well of diameter 14 m, is dug 15 m, deep. The earth taken out of its has been spread evenly all around it in the shape of a circular ring of width 7m, to form an embankment. Find the height of the embankment.
sol) volume of earth dug = Pi * r^2 * h
=> Pi * (7)^2 * 15
=> 2307.9
Volume of top platform = volume of earth dug out
Volume of earth dug out = volume of the hollow cylindrical top portion
=> 2307.9 = Pi [ R^2 - r^2] * h
=> 2307.9 = 3.14 ( (14)^2 - (7)^2) * h
=>
461.58
=> 5 = h is the height of the embankment.
*******************************************
(5) A container shaped like a right circular cylinder having diameter 12 cm, and height 15 cm is full of ice cream. The ice cream is to be filled into cones of height 12 cm, and diameter 6 cm, having a hemispherical shape on the top. Find the number of such cones which can be filled with ice cream.
sol) Given :- diameter of cylinder = 12 cm
radius(r) of cylinder = 12/2 = 6 cm
Height(h) = 15 cm
Volume of cylinder :-
=> Pi * r^2 * h
=> 3.14 * (6)^2 * 15
=> 1695.6 -----------(1)
(b) volume of ice cream cone :-
Vol of ice cream cone = Vol of cone + Vol of hemisphere
1)Volume of cone :-
Diameter of cone = 6 cm
Radius (r) = 6/2 = 3 cm
Height (h) = 12 cm
Volume of cone = 1/3 * Pi * r^2 * h
=> 1/3 * 3.14 * ( 3)^2 * 12
=> 113
2) Volume of hemisphere :-
radius(r) = 6/2 = 3 cm
volume of hemisphere = 2/3 * Pi * r^3
=> 18 * 3.14
=> 56.52
Volume of ice cream cone = 113 + 56.52
=> 169.52
Now,
Number of cones = Volume of cylinder
Volume of ice cream cone
=> 1695.6
169.52
=> 10 is the number of cones
****************************************
(6) How many silver coins, 1.75 cm., in diameter and thickness 2 mm, need to be melted to form a cuboid of dimensions 5.5 cm * 10 cm * 3.5 cm.?
sol) Number of coins = Volume of cuboid
Volume of 1 coin
volume of 1 cone = Pi * r^2 * h
diameter = 1.75 cm
radius(r) = 1.75/2 = 0.875
Height(h) = 2 mm or 0.2 cm { 1 cm = 10 mm}
=> Pi * r^2 * h
=> 3.14 * (0.875)^2 * 0.2
=> 0.4808
Volume of cuboid :-
Length ( L) = 5.5 cm
Breadth (b) = 10 cm
Height (h) = 3.5 cm
=> L * B * H
=> 5.5 * 10 * 3.5
=> 192.5
Number of coins = Volume of cuboid
Volume of 1 coin
=> 192.5
0.4808
=> 19250000
4808
=> 400 coins required
**********************************
(7) A vessel is in the form of an inverted cone. Its height is 8 cm, and the radius of its top is 5 cm, It is filled with water up to the rim. When lead shots , each of which is a sphere of radius 0.5 cm are dropped into the vessel, 1/4 of the water flows out. Find the number of lead shots dropped into the vessel.
sol) Number of lead shots = Volume of water flown out
Volume of 1 lead shot
(a) Volume of water flown out :-
Volume of water flown out = 1/4 of volume of cone
Given :- radius of cone = 5 cm and height of cone = 8 cm
Volume of cone = 1/3 * Pi * r^2 * h
=> 1/3 * 3.14 * 5 * 5 * 8
=> 209.52
Volume of water flown out = 1/4 * 209.58
=> 52. 38 cm^3
(b) Volume of one lead shot :-
Lead shot is in form of sphere
Radius(r) = 0.5 cm
Volume of one lead shot =volume of sphere
=> 4/3 * Pi * r^3
=> 4/3 * 3.14 * (0.5)^3
=> 0.523 cm^3
Now,
Number of lead shots = Volume of water flown out
Volume of 1 lead shot
=> 52.38
0.523
=> 100
Hence, there are 100 such lead shots.
**********************************************
(8) A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter 4(2/3) cm and height 3 cm. Find the number of cones so formed.
sol) volume of sphere = 4/3 * Pi * r^3
diameter of sphere = 28 cm
radius(r) of the sphere = 28/2 = 14 cm
=> 4/3 * 3.14 * ( 14)^3
=> 11,488.21
(b) Volume of cone = 1/3 * Pi * r^2 * h
diameter of cone = 14/3 cm = 4.6 cm
radius(r) of cone = 4.6/2 = 2.3
=> 1/3 * 3.14 * (2.3)^2 * 3
=> 17
Number of cones formed = 11,488.21 / 17
=> 675 cones are formed.
***********************************
(*) Optional Exercise
1) A golf ball has diameter equal to 4.1cm. Its surface has 150 dimples each of radius 2mm. Calculate total surface area
which is exposed to the surroundings ( Assume that the dimples are all hemisphere) [ 𝝿 = 22/7]
sol) given: Diameter = 4.1
Radius(r) = 4.1 = 2.05
2
Now, as the shape of golf ball is in the shape of sphere
We have,
Total surface Area of a sphere = 4𝛑r^2
Now,
Total surface Area of golf ball without dimples
=> 4*(22/7)*(2.05)^2
=> 52.78 cm^2
Given : radius of each dimple=2mm=0.2cm
In case of each dimple, surface area equal to 𝝿r^2 is removed from the surface of the ball where as the curved surface area of hemisphere 2𝝿r^2 is exposed to the surroundings
150 dimples = 150 * 𝝿r^2
150 curved surface area of hemisphere = 150*2𝝿r^2
Total surface area exposed to the surroundings = TSA of golf ball without dimples - 150𝝿r^2 + (150 * 2𝝿r^2)
=> (52.78 - 150𝝿r^2 + 300𝝿r^2)
=> (52.78 + 150𝝿r^2)
=> (52.78 + (150*3.14*(0.2)^2)
=>(52.78 + 150 * 3.14 * 0.04)
=>(52.78 + 18.84)
TSA exposed to surroundings = (71.62)cm^2
********************************************************
2) A cylinder of radius 12 cm. contains water to a depth of 20 cm. A spherical iron ball is dropped into the cylinder and thus the level of water is raised by 6.75 cm. Find the radius of the ball. [𝝿 = 22/7 or 3.14)
sol) Let radius of ball be "r2"
Given : Radius of cylinder(r1) = 12cm
Given : Height of the water level raised = 6.75cm
Given : spherical iron ball is dropped into the cylinder and the water level raised by 6.75 cm
Hence
volume of water displaced in cylinder = volume of iron ball
We know
Volume of cylinder = 𝝿r^2h
Volume of sphere = 4/3*𝝿r^3
=>
=> (12)*(12)*6.75 = (4/3)* r2^3
=> 3*(144 *6.75) = 4 * r2^3
=> 3*(972) = 4 * r2^3
=> 2916 = r2^3
4
=> 729 = r2^3
=> ∛729 = r2
=> ∛9*9*9 = r2
r2 = 9 cm
.^. radius of sphere is 9 cm.
*******************************************************
3) A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2cm. and height of the cylindrical and conical portion are 12 cm. and 7 cm. respectively. Find the volume of the solid toy. [ 𝝿 = 22/7]
sol) Given : Diameter of cylinder, hemisphere and cone is common = 4.2 cm
common radius = ((4.2)/2)) = 2.1cm
Height of Cylindrical portion(h1) = 12 cm
Height of conical portion(h2) = 7 cm
RTP :- Volume of solid toy
But Toy is consist of 3 parts if we add their volumes we can get the volume of the toy
i.e Volume of Toy = Volume of cylindrical portion + volume of hemisphere portion + Volume of conical portion
Volume of Toy = 𝝿r^2(h1) + (2/3) 𝝿r^3 + (1/3)𝝿r^2(h2)
Taking " 𝝿r^2" common
=> 𝝿r^2 (h1 + (2/3)r + h2(1/3))
=> (22/7) * (2.1)*(2.1) {( 12 + (2/3)*2.1 + 7(1/3)}
=> 22 *
=> 22 * 0.1 * 2.1*{47.2}
=> 218.06 cm^3
.^. Volume of solid toy = 218.06cm^3
********************************************************
4) Three metal cubes with edges 15 cm, 12 cm, and 9 cm respectively are melted together and formed into a simple cube. Find the diagonal of this cube.
sol) Total volume of cube formed after melting 3 metal cubes with edges 15 cm,12 cm and 9cm
We know
volume of cube = a^3
V1 = Volume with side 15cm = (15)^3 = 3375
V2 = Volume with side 12cm = (12)^3 = 1728
V3 = Volume with side 9cm = (9)^3 = 729
V1 +V2 +V3 = a^3
3375 +1728 +729 = a^3
5832 = a^3
∛18*18*18 = a
a = 18cm
We have
Diagonal of cube = a√ 3
=> 18√3
.^. Diagonal of the cube = 18√3
********************************************************
5) A hemispherical bowl of internal diameter 36 cm, contains a liquid. This liquid is to be filled in cylindrical bottles of radius 3cm. and height 6 cm. How many bottles are required to empty the bowl?
sol) Given : Diameter of hemisphere = 36cm
.^. radius(r) = 36/2 = 18 cm
Given:- Cylindrical bottle with radius =3 cm and height = 6 cm
(*) Volume of liquid in hemispherical bowl=(2/3)𝝿r^3
=> (2/3) 𝝿(18)^3 ------(1)
(*) Volume of liquid filled in one cylindrical bottle = 𝝿r^2h
=> 𝝿(3)^2*6 -----------(2)
Divide (1) / (2)
=> (2/3)
=> 2 * 18*18*18
3
9 * 6
=> 2* 6 *18*18
54
.^. 72 number of bottles required to empty the bowl
********************************************************